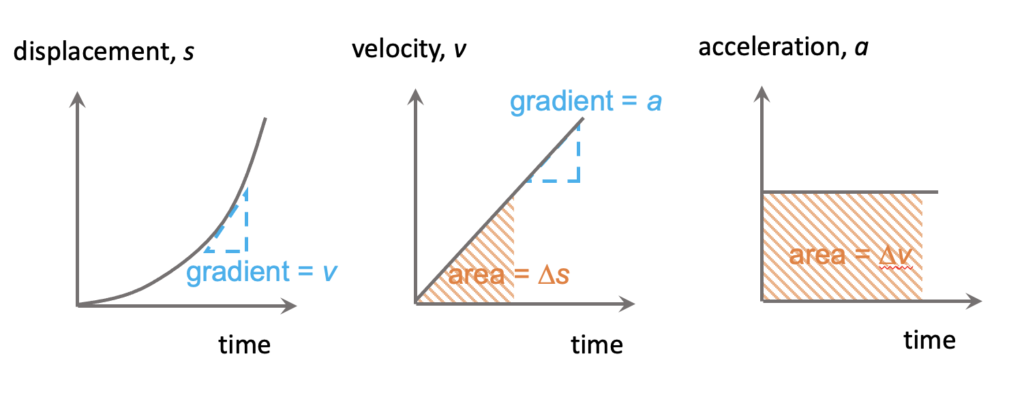
Velocity as gradient of displacement-time graph
Since velocity is defined as the rate of change of displacement, its value can be obtained from the gradient of the tangent of a displacement-time graph.
Acceleration as gradient of velocity-time graph
In the same way, acceleration can be obtained from the gradient of the velocity-time graph.
Displacement as area under velocity-time graph
Conversely, the change in displacement can be obtained from the area under the velocity-time graph. The area referred to is that which is bounded by the line in the graph and the time axis, across the width of duration of change.
If the area is found under the time-axis, the change in displacement is then given a negative sign and is associated with a decrease, as opposed to an increase, in displacement.
Velocity as area under acceleration-time graph
In the same way, change in velocity is obtained from the area under the acceleration-time graph.