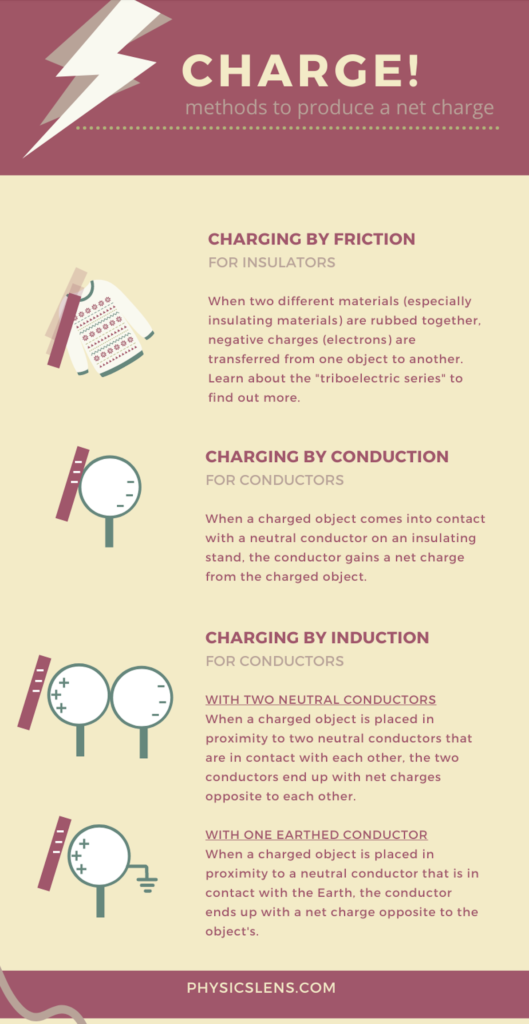
I like Canva for its ease of use and beautiful templates for creating simple infographics. This is an example of one that I did to simplify the content for IP4 Static Electricity.
the world in a different light
I like Canva for its ease of use and beautiful templates for creating simple infographics. This is an example of one that I did to simplify the content for IP4 Static Electricity.

In preparing for blended learning lessons for my JC2 students, I tweaked the Gravitational Potential applet made last year for a similar display of the electric potential between two point charges. This is a testament to the similarities between the two concepts as well as the ease of adapting a GeoGebra applet for education.
We can scaffold students’ learning using this interactive applet by asking questions such as:
To paste this applet into SLS, use the following embed code. In SLS, create a new component within an Activity within a Lesson using the “+” button. Choose Text/Media and select the button that shows “</>” or reads Embed Website/App”. Copy and paste the following codes to the box.
<iframe scrolling="no" title="Electric Potential of Two Point Charges" src="https://www.geogebra.org/material/iframe/id/z8cr66wb/width/640/height/480/border/888888/sfsb/true/smb/false/stb/false/stbh/false/ai/false/asb/false/sri/false/rc/false/ld/false/sdz/true/ctl/false" width="640px" height="480px" style="border:0px;"> </iframe>By embedding the gravitational potential distance graph for two masses, a comparison can be made between the two. This will help students draw connections between the two concepts based on the fact that the forces both follow an inverse-square law.
This is the embed code for the applet on gravitational potential.<iframe scrolling="no" title="Gravitational Potential between Two Planets" src="https://www.geogebra.org/material/iframe/id/ff55x6vr/width/638/height/478/border/888888/sfsb/true/smb/false/stb/false/stbh/false/ai/false/asb/false/sri/true/rc/false/ld/false/sdz/false/ctl/false" width="638px" height="478px" style="border:0px;"> </iframe>The concept of root-mean-square values for Alternating Currents is challenging if students are to relate the I-t graph with the Irms value directly.
They have to be brought through the 3 steps before arriving at the Irms value. This interactive applet allows them to go through step by step and compare several graphs at one time to see the relationship.
Through the interaction, students might be asked to observe that the Irms value is never higher than the peak Io.
For a complete sinusoidal current:
For a diode-rectified current:
In comparing the Irms of both currents, students can be asked to consider why the ratio of the values is not 2:1 or any other value, from energy considerations.
Worked on this earlier as I am the lead lecturer for this JC2 topic and am trying to integrate useful elements of blended learning. Do let me know in the comments if you have ideas or feedback that you would like to share.
This GeoGebra applet was modified from an existing applet to show the relationship between the pressure-distance and displacement-distance graph of a progressive longitudinal wave.
This is a 3D printed Pythagorean cup, otherwise known as a greedy cup, where if one pours far too much water or wine or whatever your greedy heart desires, all the contents in the cup will leak out through the bottom.

This is based on the design by “jsteuben” on Thingiverse (https://www.thingiverse.com/thing:123252). The siphoning effect kicks in when the water level is above the internal “tube” printed and hidden into the walls of the cup.
I printed another cup based on a more conventional design as well, but due to the wrong settings given when I prepared the gcode file, the cup was rather leaky when the water level was low. This design by “MonzaMakers” has a protruding siphon tube. (https://www.thingiverse.com/thing:562790)

Explaining how the siphon works is easier with the second cup. When the water level is lower than the highest point in the siphoning tube, it remains in the cup. When it exceeds the highest point of the tube, water begins to flow down the part of the tube leading to the opening at the bottom of the cup. The falling water column creates a suction effect and continuously draws the rest of the water in, until the cup is dry.

This pendulum-powered car is constructed using Lego Technic parts. I used mainly Lego beams to create the chassis and an “A” frame from which the pendulum is suspended. The pendulum is made of Lego beams and some wheels.
When the pendulum swings, it experiences an acceleration towards its equilibrium position. By the principle of conservation of momentum, the car experiences a change in momentum in the opposite direction. Since the acceleration of the pendulum changes its direction every half a cycle of its oscillation, the car will only oscillate about its original position if the wheels of the car are free to turn throughout the oscillation.
A escapement mechanism which consists of a beam resting on a pair of 40-tooth gears attached to the front wheels prevent the wheels from rotating in the opposite direction. This means that the car will only be moving forward during the half of the pendulum’s oscillation when its displacement is at the front of its equilibrium position and pauses during the other half.