Came across a question recently that many students answered incorrectly.
Close to the surface of the Earth the gravitational field strength is uniform. A pair of unequal masses are joined by a light, rigid horizontal bar and suspended by a string from their centre of gravity as shown. The mass M of the ball on the left is larger than the mass m of the ball on the right.
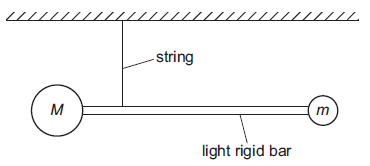
The supporting string is now cut and the system begins to fall. Air resistance is negligible.
Which statement is correct?
| A | The bar will remain horizontal as it falls. |
| B | The bar will rotate clockwise as it falls. |
| C | The bar will rotate anti-clockwise as it falls. |
| D | The bar will first rotate clockwise and then rotate anticlockwise as it falls. |
Without air resistance
This question supposes that air resistance is negligible and so the only forces initially acting on the object is weight. The answer that many students gave incorrectly as B because they assume that the larger weight acting on the larger mass will bring about a larger acceleration.
Since the object begins in equilibrium, and the acceleration of both objects is just gravitational acceleration, the bar will remain horizontal.
With air resistance
This then invites a question: What if there is air resistance?
To consider the vertical acceleration on both balls, we need to consider the net force
The volume V of a sphere is proportional to
A larger radius will imply a larger increase in V than A, and hence, a large