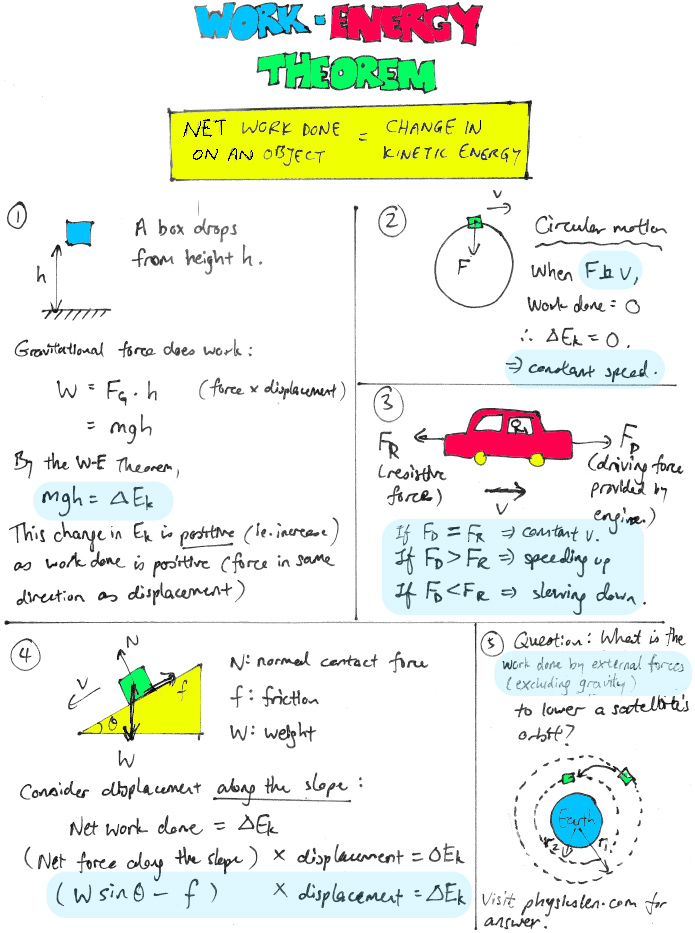
Answer to question:
Work done by external forces (excluding gravitational force) is negative.
Since [latex]E_k=\dfrac{GMm}{2r}[/latex] for satellites in orbit (where G is the gravitational constant, r is the radius of the orbit, M is the mass of the planet and m is the mass of the satellite), kinetic energy increases. Hence [latex]\Delta {E_k}=GMm(\dfrac{1}{2r_2}-\dfrac{1}{2r_1})[/latex] is positive.
Work done by gravity is positive as the radial displacement is in the same direction as that of the force. Work done by gravity = [latex]-\Delta{E_p}=-[-GMm(\dfrac{1}{r_2}-\dfrac{1}{r_1})]=2\times\Delta{E_k}[/latex]
According to the Work-Energy Theorem, net work = work done by gravity + work done by external force = [latex]\Delta {E_k}[/latex]. Hence, work done by external force = [latex]-\Delta{E_k}[/latex].